A Discussion on Math Class Placement

So it’s that time of year again. A new school year is beginning and parents are getting their child’s schedules. Getting a child’s schedule means seeing their math placement. What then follows is calls to schools and the district math office to attempt to change the math placement. It happens every year. I’ve literally had a call from a parent wanting their kid to jump from 6th grade math to algebra 2. We all want to push our children to their fullest potential, as we should, but I am always wary of skipping math content.

My own son is getting placed in a higher course. He will be skipping essentially a year and a half of math. At first, I was wary of this, so I had him work through a practice 6th grade state assessment… and he only got 4 wrong, so that eased my concern. I know, that may seem excessive, but math education is what I do and I did not want him “jumping” if he was not actually able to.
Wouldn’t he get all that math content anyway? Don’t math classes review old material a lot anyway? The answer to that is no. They used to, but they never should have. You see, the U.S. math curriculum had a reputation for being “a mile wide and an inch deep.” We covered a lot every year (too much), a lot of the same math concepts, and didn’t go very deep with it. Our math courses taught breadth. This is part of why we, in general, do not do to well in math and have prevalent math phobia.

Most of the world that performs well in math does the opposite. They cover less math each year but teach the concepts with great depth. This means, each year of math builds upon the last year. Math instruction isn’t repeated. It is extended to greater depth each year. So if you skip a year, you essentially skip a year of content. The only way you should skip is if you actually know or can do the math you skipped.

This needed change came with the common core push (even states that didn’t adopt common core standards adjusted their standards similarly). I won’t get into a common core advocacy discussion here; I do that inadvertently in a lot of my math posts that discuss the reason math is now taught the way it is. I will say though that if you read the standards, they tell you what to teach, not how to teach it. That’s what standards do. I’ll leave it at that for now.

Back to my original point. I firmly believe you never hold a child back who is capable. If a child is ready to jump to higher math and can handle it, by all means let them. BUT… but… only if they can. Do not skip content for the bragging rights of a child being in a higher course. They struggle. Sometimes they fail. I’ve taught these kids. Parents pushed them ahead. They are stressed in class. They cry at their lockers… sometimes in class. In 20 years of teaching math, I have had these students. Now I’m not one that likes to “lose,” so of course I put the time in to help them and try to help them succeed. But, they probably should not have been jumped ahead.

There is no shame in being on grade level. A child that is on target should be in their grade level course. We should not push children ahead of where they are ready to be. It hurts more than it helps. It can create more misunderstanding and more math misconceptions. The conceptual gaps become greater. And if the child should be advanced, the teachers will typically notice and recommend. Typically.

There is also a lot of talk about pathways in mathematics right now. Developing strands of courses that children can take in high school that will provide them with the correct mathematics background for future college and career readiness. The landscape of high school mathematics will likely be shifting a bit over the next decade. I’ll talk more about this in a future post, but I mention this to say there really is no need to push children into advanced classes faster to reach certain “goal” courses before high school graduation - courses like calculus. We often think in terms of the traditional track towards calculus, but realistically, not every child needs to study calculus. More honestly need further coursework in statistics as that is more applicable to many career fields. A child who is on grade level will have the opportunity to take the math courses they need to take without prematurely advancing.

So, all this to say, before you jump to push for higher math placement, take the 11th commandment to heart: thou shalt not jive thyself. Only push for a higher placement if the child REALLY knows the math they would be skipping and you really think the teacher “missed” something. Conversely, if a school recommends higher placement, be sure a child is ready. Advancing a child before they are ready causes them to completely miss math content (maybe foundational content), and will cause them confusion, anxiety, and unnecessary struggle for years to come.

To Mnemonic or Not To Mnemonic: The Problem with PEMDAS

I’m sure by now we are all sick of seeing all of math problem posts that circulate around all of the social media sites pretty regularly. They usually begin with “Only x% of people get this correct!” What follows is a reasonably simple orders of operation problem which people in the comments argue about. The arguments typically center around the rules they learned for the orders of operation. 

“Well, according to PEMDAS...”

Ugh! And the debates over the meaning of this horrible mnemonic tool then ensue. The interesting thing is, based on what country you are born in, you may have learned a different mnemonic for performing computation. PEMDAS isn’t universal. If you are in Australia, India, or the United Kingdom, you may have learned BODMAS. If you are in Canada or New Zealand, you have have learned BEDMAS. Different people learn a different mnemonic, and these mnemonic tools are a big part of the reason why people have misconceptions about the order of operations.

I’m going to focus on PEMDAS for this discussion. I’m sure most everyone that leaned it in grade school remembers what PEMDAS stands for: 

P = parentheses,
E = exponents,
M = multiplication,
D = division,
A = addition, and
S = subtraction.

The point of this tool being that it helps you remember the order in which the perform the operations from left to right. The problem is, if you take the tool literally, you will perform the calculations incorrectly. PEMDAS has given many the misconception that you perform multiplication first, then division, then addition, and lastly subtraction. This is not correct though.

Why is this not correct? Because, in reality, those four operations are really just two operations. Multiplication and division are inverse operations, and addition and subtraction are inverse operations (with subtraction and division kind of being imperfect inverses, but that’s another conversation). As a result, each pair of operations should be treated one in the same. 5 - 2 is equivalent to 5 + (-2) and 6 ÷ 2 is equivalent to 6 × ½. There is an additive equivalent for every subtraction expression and there is a multiplicative equivalent for every division expression. Therefore addition and subtraction should be performed together and multiplication and division should be performed together.

So, the correct orders of operation are actually:

1. Grouping symbols,
2. Exponents, 
3. Multiplication OR Division (from left to right)
4. Addition OR Subtraction (from left to right)

We can apply it to the problem below as an example. Notice in the final steps, we perform the subtraction before addition, because when moving left to right, subtraction comes first.

This is one reason why I am generally not a fan of teaching mnemonics in this manner. Although these tools are developed with well meaning, they can bring upon misconceptions or even fall short. 

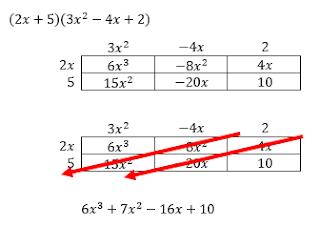
Take FOIL as another example. It’s used to teach polynomial multiplication. You multiply the first terms (F), then the outer (O), then the inner (I), and then the last terms (L). This seems like a solid way to learn the sequence of multiplication steps needed before finally simplifying the polynomial, but the problem is, it only works for multiplying two binomials. If a student learns FOIL and is then presented with multiplying a trinomial by a binomial or with multiplying two trinomials, they may be at a loss.

A better approach would be to build off of the their understanding of the Distributive Property and extend it to multiplication with polynomials. When multiplying polynomials you are performing the distributive properly multiple times in sequence. We could also return to the array method that students learned as a conceptual stepping stone in elementary school which is an excellent visual to make sense of the algebraic nature of polynomial multiplication. Teaching it in either of these manners removes the restrictions that FOIL has and expands it to cover all polynomial cases.

Returning to PEMDAS, similarly, ensuring students understand the inverse and interrelated relationships of addition and subtraction and multiplication and division would help to alleviate misconceptions regarding these arithmetic processes. Exploring the conceptual aspects of the orders of operation would be far better for understanding than just memorizing a problematic mnemonic. Or, if a mnemonic is necessary, perhaps something more reliable, like PEMA (Please Eat More Avocados??). Focusing on the stronger operations of multiplication and addition (reinforcing that subtraction is a form of addition and division is a form of multiplication).

But, in the end, these mnemonics are there to aid procedural skill, and as I have mentioned before, conceptual understanding is more powerful than memorizing procedure. Focusing on truly understanding the concept will typically alleviate any need for tricks to help memorize procedure.

Don't Memorize Formulas! Visualize

It's been a minute since I've gotten "mathy," so I felt it was time to dig back into it a little. Last time, I talked about using the structure of expressions as a means to make sense of the math rather than memorizing formulas. I kind of jumped a head and got into circles since it was right around Pi Day, so let's take a few steps back. This will be kind of a "part 2" to that entry. 

Continuing with the theme of not memorizing formulas, let's look at some math "common sense," for lack of a better way of saying it. If you draw things out, you can often visually see the math behind formulas. The formulas weren't magically plucked out of the air. The formulas you come across in math tell a story. They describe exactly what needs to be done. In the case of area formulas, they tell you how to put the pieces of the shape together to figure out how much space is inside of the shape. So let's look at an example of this "common sense," the area of a triangle.

Why is the area of a triangle 1/2bh (one half times base times height)? Well, let's look at a rectangle. The area of a rectangle is its length times its width (l ∙ w). If a rectangle is 4 units long and 3 units wide, drawing this array out will show that there are 12 square units inside the shape, or 4 ∙ 3 = 12. Most students have no problems with the area of a rectangle; it's a pretty easy concept to grasp. Before moving on, a slight note: mathematically we don't often use length and width for a rectangle. Instead of length, we'll refer to base, and instead of width, we'll refer to height. So a rectangle would be base times height, or b ∙ h.

If we were to draw a diagonal down the center of the rectangle, we would split it into two triangles. Each triangle would be half of the area of the original rectangle. This is why the area of a triangle is one half times the base times the height. You are literally cutting the rectangle in half. So the area of the triangle with the same base and height as the rectangle is half the area. This is a fairly easy notion for students to grasp visually; it's almost "common sense" when you look at it drawn out. So again, rather than just memorizing formulas, add meaning to it.

Once you understand the area of a triangle, you have a terrific tool at your disposal. You can visually break down so many other areas using triangles. From there, you can build the formulas for the areas using the triangles. I never had students memorize any perimeter, area, or volume formulas. I had them construct the formulas by "deconstructing" the figures visually. Two examples are shown below.

You can divide a trapezoid into two triangles. Each triangle has the same height and the bases are the "top" and "bottom" of the trapezoid. Add the areas of these two triangles together and you have the area of the trapezoid. I would have students do this and label each part with a variable and build the formula for the area of a trapezoid for themselves just like shown above. A similar process can be done for a rhombus. If you divide it into two triangles, you see that the two triangles share the same base, which is the horizontal diagonal of the rhombus. The two heights of the triangles together form the vertical diagonal of the rhombus. When you put it all together, you see that the area of a rhombus is half of the product of its two diagonals, which is actually the formula for the area of a rhombus. Students often find building this formula kind of fascinating. You can find the area of pretty much any polygon by dividing it up into a bunch of triangles and then adding up the areas of the triangles; doing this algebraically with variables will typically yield the formula for the area. 

Building the formulas this way is far more meaningful. Just memorizing a formula doesn't allow a student to gain any meaning to it. They have no understanding to attach the formula to. So, they often just forget it. It has no meaning. When a student constructs a formula by visually breaking it down like this, they see what each part of the formula means and make sense of it. I find that students often remember the formulas once they attach meaning to them like this. Or... if they forget the formula... since they have a concept of where the formula came from, they can rebuild it. Or... at the very least... they can divide the shape up into triangles and find all the areas and add those up. Visualizing like this is just another way of understanding or seeing structure, which is a very important part of mathematics. So again, don't memorize.... visualize... see the structure.... make sense of the math.

Don't Memorize Formulas! Understand Their Structure

I hate the notion of memorizing formulas. Formulas do not need to be memorized. They need to be understood. A major focus of the high school mathematics standards is the idea of “seeing structure in expressions.” This key idea extends down into the lower grades as well. What does this mean? It means you understand what each piece of the expression or equation means or does. You can read an expression or equation almost like you would read a sentence.

In honor of π Day earlier this week, let’s use some of the relationships of π with circles to illustrate this. If we think back to how we learned circumference and area of circles back in school, there’s a good chance it came down to being told the formulas straight out and then shown how to plug values in to use the formulas in a few examples. Then we were given a ridiculous amount of skill based problems to practice. This is a horrible way to teach this... or, well... anything in mathematics really. Students should figure out formulas and concepts on their own through exploration and sense making... with the teacher questioning and guiding.

Let’s look at circumference. Give a class of students a bunch of circular objects. They measure the diameters and record them. They then wrap string around the objects, cut the length, straighten them out and measure. Now they compare the circumference with the diameter. What do they notice? Through examining all the data collected and some discussion, they notice that the circumference is always a little more than 3 times the diameter. As they look more closely at the data, they eventually get more specific and see that the circumference is precisely π times more than the diameter. The diameter can be wrapped around the circle π times. They then translate that sentence into a formula, C = πd.

Rather than being told, they make sense of the relationship and build the formulas themselves. And since they built it themselves, they don’t need to memorize it. They had an “a-ha”! They understand the concept and the formula is just a mathematical way of showing the concept. If you get the meaning, you don’t need to memorize formulas... because the idea makes sense.

Similarly, they can explore area of circles. They are directed to make a square out of the two radiuses (or radii) as shown in the one picture. They find the area of the square (radius times radius) and then painstakingly find the area of the whole circle by counting grid squares. They compare these two areas and once again see that the area of the circle is π times the area of the square. The square made by the radii fits into the circle exactly π times, hence A = πr².

Memorizing is typically never necessary if there is sense making. If you understand the concept, you can build the formula, because you understand the relationships and structure.

Fair Sharing: Developing Division

 Zoe, my daughter in Kindergarten, was working on a math problem yesterday. She had to share 8 cookies between 2 people. She split each cookie in half. Was she wrong? Technically no. But I asked her, “if you and I were going to share 8 cookies, would you break each cookie in half and share them that way?”

“No, I wouldn’t do that,” she replied.

I pulled out 8 double sided counters (those round little red and yellow chips). “Show me how you would share the 8 cookies between us.”

“One for me. One for you. One for me. One for you...” She continued until she had two piles of 4. “We each get 4 cookies!”

This is a concept known as fair sharing. This is how division begins in early grades. In many cases, children may even learn this before grouping (multiplication), as sharing is more of a pressing notion than making groups. 😁

When time comes to really dig into division later in elementary school, children have had plenty of work with place value and multiplication under their belts, so their first experience may look something similar to the first picture. Can they divide by subtracting “groups” from the number. For 1,375 ÷ 5, they likely know that 5 × 100 = 500 and 5 × 10 = 50, from work with multiplication and place value, so they may start by removing those groups first. Work with money in prior grades will likely bring 5 × 20 = 100 to mind as well. Eventually, when they add up that far right column, they see that 1,375 ÷ 5 = 275. This looks like a real ugly and clunky approach, but it allows them to make sense of the whole idea of division/long division. Again, we don’t want memorization of mindless procedures, we want sense making . This builds toward the long division procedure (and builds mental math in the process).

Eventually we work toward methods like those shown below, more “formal” long division algorithms. Now we are attempting to find the largest possible grouping to subtract out each time. Students will likely already be working toward this as they gain more “finesse” through exploration with the prior methodology (picture 1). I personally prefer the “box method." It takes up less paper and I think it allows for a better visual understanding of the place value aspects of division.

What's the Deal with Multiplication Arrays?

Let’s talk multiplication. I’ve seen people losing their minds over seeing children using the multiplication array on the bottom, and there is no reason.

If we go back to the early grades multiplication is often introduced through grouping. Children concretely group things to multiply. “How many marbles are there if we have four groups of three marbles?” Eventually we want to move to more representational and abstract approaches, and like with subtraction, we want to build off of the heavy place value emphasis from Kindergarten and First Grade. That’s where that multiplication array comes in. Children know that 47 is 40 + 7 and that 23 is 20 + 3. Multiplying each of the pieces is much easier since they have worked with single digit “times tables” and multiplying by 10s is also easy. And the four numbers can be easily added. 40 x 20 = 800, 40 x 3 = 120, 7 x 20 = 140, 7 x 3 = 21, 800 + 120 + 140 + 21 = 1,081. Comparing to the standard algorithm at the top, the square array is the same approach, but it is easier for the children to see what is actually happening. It’s a conceptual stepping stone to the standard algorithm so they can make sense of multi-digit multiplication using place value.

The arrays also help build mental math, which as I said before, is an important goal. With a problem like 25 x 12, starting with the array allows them to get to point of doing something like “25 x 12 is 25 x 10 and 25 x 2. 25 x 10 = 250 and 25 x 2 = 50. 250 + 50 = 300.” Again, looks like a lot written, but takes bit a few second in your head. The array visual helps improve mental multiplication.

Lastly, progression in math is important. Eventually students get to algebra and “more abstract” math in later grades. Arrays are used to multiply polynomials, for example, as in the last pic. Having already seen and used arrays for multiplication allows the student to make a conceptual connection to the algebra. They see multiplication is the same in all facets. We’re building coherence.

These arrays are not new math or a new way to multiply. They are a tool for understanding and visualizing the concept of multiplying and for building mental math. (And they’ve actually been around a long time.)

Is the Standard Algorithm Always Best?

I always see comments from parents on the way their children learn math. The way math is taught now should look rightfully different. The way math was taught in the past focused too much on memorizing procedures and steps. Conceptual understanding and application often took a back seat for procedural fluency. All three are equally important in math, but conceptual understanding and application should be prioritized first in my opinion. If someone understands those two pieces well, the procedural piece will likely come easily... or even on its own without instruction on it. The standard procedure we were all taught isn’t even always the best way.

An example... borrowing. Who likes borrowing in subtraction? It’s annoying. If someone has number sense, a conceptual understanding of how numbers work, it’s unnecessary. Take the example below. 1000 - 843. The whole borrowing from zero thing that was always taught is cumbersome. That’s a lot of borrowing and time to subtract with the standard algorithm. Let’s ponder the idea of differences for a moment. 10 - 6 = 4, 9 - 5 = 4, 8 - 4 = 4, 7 - 3 = 4. What do we notice? When both numbers are reduced by 1, the difference is still 4. Shifting both numbers by the same amount preserves the difference! So forget borrowing; use that number sense. Instead of 1000 - 843, let’s reduce both by 1 and do 999 - 842 instead. Much faster! 157!

Also, those arrow strings you may see elementary students doing. We in math education call that “counting on.” It is a way to visualize subtraction using all the place value they worked with in Kindergarten and first grade, and it’s also how the brain can easily process subtraction mentally. Part of the goal is to build better mental math. Now it looks like a lot on paper because children need to write it out and visualize it at first. But over time they can REALLY quickly in their heads do... “843 plus 7 is 850. 50 more is 900. 100 more is 1000. So, 100 + 50 + 7 is 157.” That seems like a lot written out, but that takes mere seconds in one’s head.

The “standard algorithm” isn’t always the best approach. Conceptual number sense is key.